Polar topology

In functional analysis and related areas of mathematics a polar topology, topology of $\mathcal{A}$ -convergence or topology of uniform convergence on the sets of $\mathcal{A}$ is a method to define locally convex topologies on the vector spaces of a dual pair.

Definition

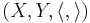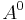Given a dual pair $(X,Y,\langle , \rangle)$ and a family $\mathcal{A}$ of sets in $X$ such that for all $A$ in $\mathcal{A}$ the polar set $A^0$ is an absorbent subset of 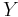$Y$ , the polar topology on 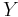$Y$ is defined by a family of semi norms 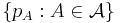$\{p_A�: A \in \mathcal{A}\}$ . For each $A$ in $\mathcal{A}$ we define

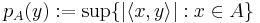$p_A(y):=\sup\{\vert \langle x , y \rangle \vert�: x \in A\}$ .

The semi norm $p_A(y)$ is the gauge of the polar set $A^0$ .

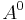Examples

a dual topology is a polar topology (the converse is not necessarily true)
a locally convex topology is the polar topology defined by the family of equicontinuous sets of the dual space, that is the sets of all continuous linear forms which are equicontinuous
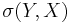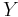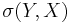Using the family of all finite sets in $X$ we get the coarsest polar topology $\sigma(Y,X)$ on $Y$ . $\sigma(Y,X)$ is identical to the weak topology.
Using the family of all sets in $X$ where the polar set is absorbent, we get the finest polar topology $\beta(Y,X)$ on $Y$

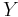
Notes

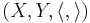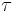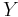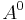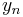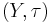A polar topology is sometimes called topology of uniform convergence on the sets of $\mathcal{A}$ because given a dual pair $(X,Y,\langle , \rangle)$ and a polar topology $\tau$ on $Y$ defined by the gauges of the polar sets $A^0$ , a sequence $y_n$ in $(Y, \tau)$ converges to $y$ if and only if for all semi norms $p_A$

$\lim_{n \to \infty} p_A(y_n - y) = \lim_{n \to \infty} \sup_{x \in A} \vert \langle y_n - y, x \rangle \vert \to 0$

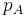
Or, to put it differently, for all sets 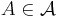$A \in \mathcal{A}$

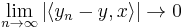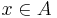$\lim_{n \to \infty} \vert \langle y_n - y, x \rangle \vert \to 0$ converges uniformly with respect to $x \in A$ .